how to find experimental probability

What is the Difference Between Theoretical and Experimental Probability?

Theoretical and Experimental Probability

FAQs Practice

Frequently Asked Questions

Q

What is theoretical probablilty?

A

Theoretical probability is the likelihood of an event occurring. The formula for theoretical probability is:
P(A)=\(\frac{\text{number of acceptable outcomes}}{\text{number of possible outcomes}}\)
Ex. The probability of rolling a 2 on a standard 6-sided die is \(\frac{1}{6}\). There is one acceptable outcome and a total of six possible outcomes.

Q

What is experimental probability?

A

Experimental probability is an estimate of the likelihood of a certain outcome based on repeated experiments or collected data.
Theoretical probability is based on what should happen, while experimental probability is based on what actually happened.

Q

What is the difference between theoretical and experimental probability?

A

The difference between theoretical and experimental probability is that theoretical probability is based off what should happen and experimental probability is based off what has already happened in experiments or trials.

Practice Questions

Question #1:

What is the theoretical probability that an even number will be rolled on a dice?

\(\frac{2}{6}\)

\(\frac{3}{4}\)

\(\frac{1}{2}\)

\(\frac{6}{3}\)

Show Answer

Answer:

The correct answer is C: \(\frac{1}{2}\).

There are three even numbers and three odd numbers on a dice. Our options for rolling an even number are 2, 4, 6 and our options for rolling an odd number are 1, 3, 5. There are \(\frac{3}{6}\), or \(\frac{1}{2}\), ways to roll an even number.

Hide Answer

Question #2:

If you roll a dice 36 times, how many times would you expect to roll a 4? Use theoretical probability to determine your answer.

3 times

4 times

5 times

6 times

Show Answer

Answer:

The correct answer is D: 6 times.

Theoretical probability tells us that there is only one way to roll a 4 on a die. Since a die has six sides, this represents \(\frac{1}{6}\). If we roll a dice 36 times, we can expect to roll a 4 one-sixth of the time. One-sixth of 36 is 6. We should theoretically roll a 4 six times.

Hide Answer

Question #3:

Flipping a coin 8 times should theoretically result in 4 heads and 4 tails. However, when Sam runs this coin flipping experiment, he ends up flipping 2 heads and 6 tails. Express this experimental probability of flipping a tails in the form of a percent.

Show Answer

Answer:

The experimental probability of flipping a tails is 6 out of 8 for this particular scenario. 6 out of 8 can be expressed as the fraction \(\frac{6}{8}\) which can be simplified to 34. From here we can multiply the numerator and denominator by 25 in order to have a fraction that is out of 100. This leaves us with \(\frac{75}{100}\) or 75%.

Hide Answer

Question #4:

Max is using a circular numbered spinner that has 20 equal sections. What is the theoretical probability that the spinner lands on a number greater than 14? Express your answer as a percent.

Show Answer

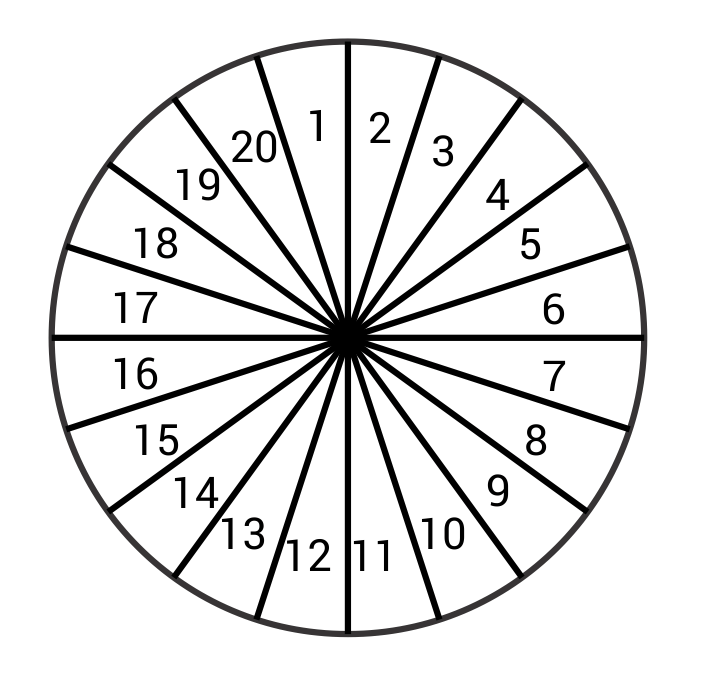
Answer:

The correct answer is A: 30%.

Numbers that are greater than 14: 15, 16, 17, 18, 19, 20. There are 6 numbers larger than 20, so the probability can be represented as \(\frac{6}{20}\) or simplified as \(\frac{3}{10}\). \(\frac{3}{10}\) as a percent is 30%.

Hide Answer

Question #5:

Sophia sets up an experiment using a deck of 52 cards. She selects a card at random, records the suit of the card, and then replaces the card in the deck. Sophia recorded her results in the table below. What was the experimental probability of selecting a diamond?
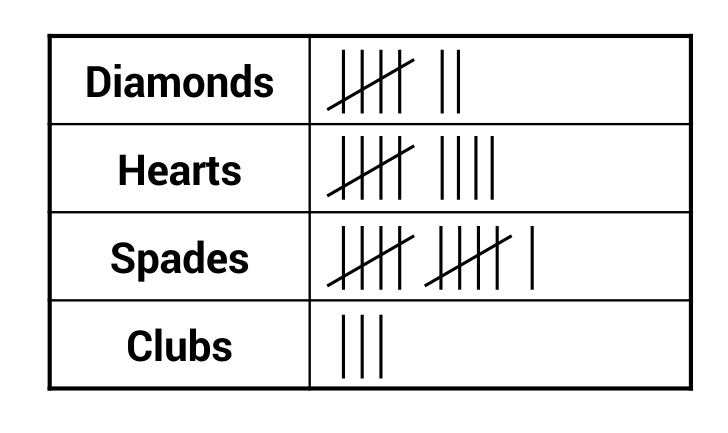
probability suits

\(\frac{7}{52}\)

\(\frac{7}{30}\)

\(\frac{14}{20}\)

\(\frac{11}{30}\)

Show Answer

Answer:

The correct answer is B: \(\frac{7}{30}\).

During Sophia's experiment she selects and replaces 30 cards. The number of diamonds selected is 7, so we can set this up as the fraction \(\frac{7}{30}\). 7 out of 30 selections were diamonds.

Hide Answer

444349 466775